Focal Length and Depth of Field

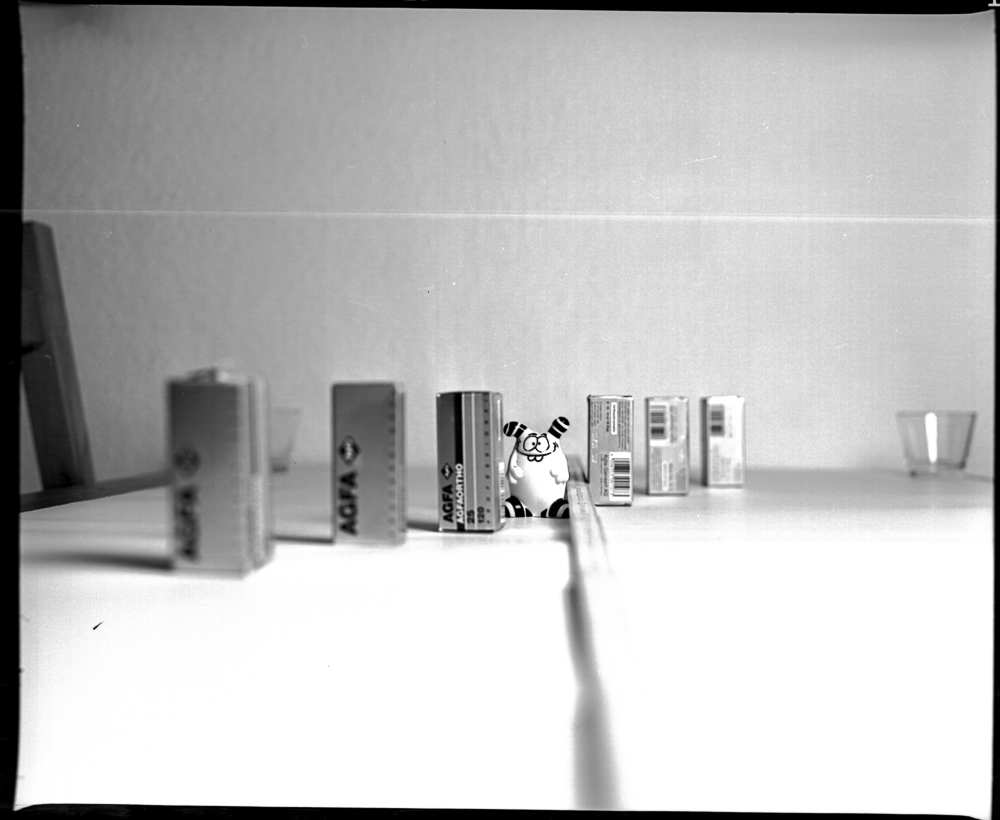
You have probably been confused by discussions on the web about crop factors, full frame versus APS-C versus medium format and why the pros use full format while crop factors are for wimps.

Well, I want to add to that confusion…

So, what is the discussion all about, besides egos and masturbation over who has the larger equipment? It’s depth of field and sensor noise. Sensor noise is something which is more or less controlled nowadays, current APS-C Sensors provide very usable images up to 6400 ISO, so the argument goes more or less away (Full frame sensors have bigger pixels, which create less noise, but this turns more and more academic, as the noise in APS-C cameras gets lower and lower…). Remains depth of field: Depth of field describes the region where the image appears sharp.

An image appears sharp when the circle of confusion of the image (ah, now you are confused in circles!) is smaller than the resolution of the human eye. Any point of the object to be photographed will be translated to a small circle in the image. So if you have a point like light, it will be a small circle in the image, as the resolution of a lens is not perfect. If the circle is smaller than your eye can resolve, you think it’s a sharp image.

If you do the math (the derivation of these formulae is left as an exercise to the reader), you get the following distance for the point closest to the camera which is considered sharp:

$$ D_n = \frac{s (H-F)}{H+s-2F} $$

and this for the point furthest from the camera

$$ D_f = \frac{s(H-F)}{H-s} . $$

H is the hyperfocal point, calculated as

$$ H = \frac{F^2}{f\times c}+F ,$$

F is the focal length of your lens, f the aperture, s the distance to your subject and c the maximum size circle of confusion considered sharp (about 0.03mm for 35mm film).

In case you are too lazy to do the math, there are also online depth of field calculators.

What you see here is that the film/detector size does not enter the equation directly. It enters only via the maximum size of the circle of confusion. If you have a larger film, you do not need to enlarge your print as much as if you have a smaller film. Therefore the maximum allowed circle of confusion can be larger.

What can we do now with these formulae? Well, calculate of course…

Let us have a look at various cameras with a „normal lens“, which is a lens where the focal length is roughly equal to the diagonal of the film/sensor, leading to a field of view of about 53°. This field of view corresponds to a viewpoint which is more or less similar than the human eye (if you take a SLR with a normal lens and look with one eye through the viewfinder and the other directly at your photographic object, you see that it appears more or less the same size with both eyes).

Assume we have an object 3ft away from the camera and we take a picture with a 6×7 camera, like a Mamiya RB67. We use a „normal lens“, which in this case is a 90mm lens, set to f6.4. Calculating the depth of field we see that everything between 2.9ft and 3.11ft is sharp. (As sharp as things can get on a badly developped film which had its due date 15 years ago scanned on a relatively cheap flatbed scanner…)

Now we change camera and take the same picture with a Canon EOS50e (35mm film), using a 50mm lens. The field of view is identical (more or less), but to get the same depth of field, we need an aperture of 3.6.

And now we get digital and do the same picture with a Canon EOS50D (APS-C Sensor), using a 35mm lens, also giving the same field of view. We now need an aperture of 2.8 for the same depth of field.

All the images are not cropped and not really postprocessed (only white balance and brightness adjusted, the rest is as out of the camera/scanner). From this exercise we are led to

Which in itself is nothing bad, as you have a wider aperture on the lens, you can now also use a shorter exposure time. The negative part is: lenses with wider apertures are harder to develop and manufacture, so they are usually much more expensive. (If you check these values above, they have been carefully chosen so that I end up with f2.8 for the APS-C camera, so I can take the picture with the lens I have).

So, now you say „Ah, so it’s only a question of money to get the same image with an APS-C Camera than with a medium format?“ – Well, yes and no. While you can create an image with the same depth of field, there is another difference. Let’s get back to math (well, actually physics, but for the non-scientist reader it’s all the same boring math-stuff):

For a simple thin lens (which we assume to represent our highly expensive 2kg of finest japanese or german glass) the magnification factor can be calculated as

$$M=\frac{i}{o},$$

where i is the image distance and o is the object distance to the lens. With the lens equation

$$\frac{1}{o}+\frac{1}{i}=\frac{1}{F}$$

with F as focal length, we get

$$M=\frac{1}{\frac{o}{F}-1}$$

From which we can calculate the following: let’s assume we have an object at 6ft from the lens and one at 12ft. For an APS-C camera with a 35mm lens, the object at 12ft will be 0.45 times the size of the one at 6ft. For the 35mm camera with a 50mm lens, it will be 0.42 times the size and for the 6×7 camera with the 90mm lens it will be 0.35 times the size. And this leads us to

As you see, the difference between APS-C and „Full Frame“ is negligible. The difference to a 6×7 camera is a tiny little bit larger and maybe this camera might create a different image impression than the full frame.But I doubt that anyone would notice…

And if you noted that I really oversimplified the calculations, you get two bonus points…

Conclusions

So, what do we learn from all this? To get the same depth of field as a full frame or medium format camera, you need a lens with a larger aperture, which is more expensive and probably has a worse resolution than the lens you would need on the full frame or medium format camera. If you want your image sharp and you do not want a razor thin sharpness zone, you are perfectly fine with a small sensor. If you need short exposure times with a relatively narrow depth of field, a small sensor is also your thing. If you need images which are as sharp as possible in a narrow region and are bokeh-ing around everywhere else, you need to get an as large sensor/film as possible.

But in general the difference is more or less negligible. If you have a fast lens on an APS-C camera, you can get similar images as with a slower lens on a full frame. Only when you get to the extremes, you will notice a difference.

There are also small differences in the perspective of the various focal length. So an image created by a 50mm with a full frame sensor and an image created with a 35mm with an APS-C Sensor will look slightly differently when you check the relative size of objects with each other. However I doubt that you see this difference with the naked eye – you probably need to go and measure with a ruler in a direct comparison. You might notice the difference with a large or medium format however.

The most notable difference here is that a 35mm film scanned on a flatbed scanner looks worse than a 6×7 film scanned on a flatbed scanner and both look worse than a digital camera. But I think you got the general idea of what I was trying to say.

And now go off and spend more money on equipment instead of taking pictures, that’s what differentiates the Pros from the wimps…